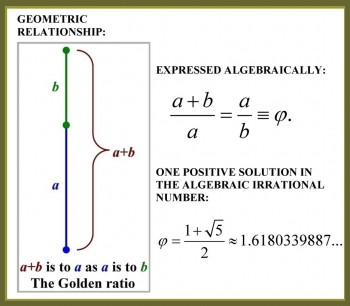
Fig 1: The golden section constitutes a line segment divided according to the golden ratio. The total length a + b is to the longer segment a as a is to the shorter segment b.
In this article: Golden section and golden rectangles, the harmonic series and the model of its ideal behavior simplified, equal temperament and just intonation and a description of the beat frequency conflict produced by the deviations between simultaneous sounding of harmonic partials and prime frequencies and how it affects the tone and timbre of the guitar. Read to the end for some cool videos demonstrating the properties detailed here.
Beginning in the Renaissance, a whole rebirth of literature occurred that encouraged the use of the golden ratio (see note 1) in the dimensional relationship of many objects that aimed to possess an aesthetic and pleasing form.
These objects include buildings and statues as was done in the ancient period (see note 2) as well as forms found in paintings, books, instruments etc., and this repetitious practice of applying either the golden section or the golden rectangles to the forms of the constructed objects engaged most branches of the arts and sciences.
The golden section is a ratio based on the Greek letter phi (see note 3) written usually in lower case (φ) (=1.618033988749895…). It is also known as the Golden Mean, Golden Ratio and Divine Proportion as defined by Renaissance artists. Fig. 1. illustrates the geometric relationship and the algebraic expression that defines this constant (see note 4).
The golden ratio is expressed as the golden section (see note 5) of the line segments and also in the form of the golden rectangle, in which the ratio of the longer side to the shorter is the golden ratio. Both these forms involving the line and the two dimensional rectangle have been used extensively at least since the Renaissance by many artists and architects in defining the proportions of their work.
Fig. 3. illustrates the golden section found in line segments a and b and also the three golden rectangles found in “The Kertsopoulos mathematical model of the guitar” as shown in Vol. 1 of 7 of the series. Also, in Fig. 4. the additional six golden rectangles are shown.
When a sound includes vibrations of one and only frequency, is named a simple tone. Most musical instruments produce compound-complex tones, each tone producing a combination of many frequencies, which however, are comprehended by the human perception as a unity that forms one whole sound and this unique function of the human hearing conception has been named fusion.

Fig 3: The golden section and the three golden rectangles found in “The Kertsopoulos mathematical model of the guitar”.
The lowest frequency within the compound tone is called the fundamental or prime and by being generally the loudest, conveys the sense of pitch of the tone, ex: C,D,E etc.
The other frequencies of the compound tone are called harmonic overtones or harmonic upper partials. These two definitions clarify the relation that exists between the fundamental or prime frequency and all the other frequencies or simply harmonics.
Their relation with the fundamental is expressed in whole-number ratios. This relation of the harmonics that defines the synthesis of every musical compound tone is called harmonic series.
Every harmonic overtone has a simple ratio relation with the prime. The ideal frequency of each overtone being an integer multiple of the prime, depending on the ordinal number the specific overtone holds within the series, ex: the fifth harmonic of E = 82.4 Hz is 82.4 x 5 = 412 Hz = g’#.
The word ideal is very important to be noticed because in real performance conditions of an instrument there are serious deviations occurring in all its musical sound productions in regard to the expected ideal frequency of each harmonic in its relation with the fundamental.
Of course, the ideal model of the harmonic series defines specific harmonics that will deviate upwards or downwards from the expected ideal frequency but in actual performance conditions all instruments have various deviations even on harmonics that the ideal model defines as stable.
The ideal behavior of the harmonic series remains the same for all musical tones. Every instrument though has its distinct sound, because of the fact that it deviates variably in its own peculiar manner from the ideal model itself. There are many additional factors involved that can be found on the specific presence in relative percentage value of each harmonic of the compound tone or the specific waveform outline of each harmonic or the noise presence that might occur in-between the harmonics.
A full acoustical Fourier analysis of a recorded complex guitar sound shows with a great accuracy all these deviations that occur and we will show further on in this series various Fourier analysis done on three different guitars.
All the harmonic overtones and including the prime, are called generally: harmonic frequencies or simply harmonics, the 1st harmonic being considered the prime and the 2nd harmonic being the 1st harmonic overtone.
For reasons of clarity the term prime will be used in this series and the term “harmonic” for the harmonic overtone, the 2nd harmonic being considered to be the 1st harmonic overtone.
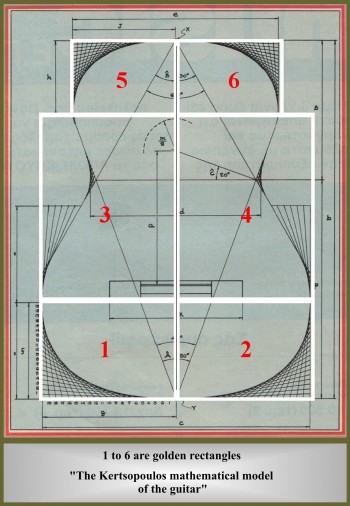
Fig 4: The six additional golden rectangles found in “The Kertsopoulos mathematical model of the guitar”
It can be observed, that the just ratios which form the common traditional interval names of the diatonic system, evolve directly from the whole-number ratios existing within the harmonic series. Also, because of their association with the intervals found in the harmonic series, intervals such as 2:1, 3:1, 3:2, 4:3, 5:3 are often called natural intervals. A perfect fifth that is expressed with the ratio 3:2 is formed by the third and second harmonic of the series.
By dividing the numbers 2,3,4,5,6 with the corresponding numbers 1,2,3,4,5, we obtain the just ratios on the left that also give the equivalence of the traditional interval names and in each parenthesis the interval inversions are shown in fig. 5:
Harry Partch distinguishes between the 16:9 “small just ‘minor seventh’” and the 9:5 “large just ‘minor seventh’”. Also, the 16:15 ratio concerning the semitone in its most common form in just intonation takes a different value in 12-tone equal temperament, which is a form of meantone tuning where the diatonic and chromatic semitones are exactly the same, due to the unbreakable circle of fifths.
In equal temperament used by the guitar’s fret locating idiom, each semitone is equal to one twelfth of an octave. Every frequency is multiplied by 1.059463094 (1.05946 is regarded adequate) to reach the frequency of the next higher semitone. This ratio being the twelfth root of 2 () is equivalent to 100 cents but it is 11.7 cents narrower than the 16:15 ratio found in just intonation.
These are basic discrepancies occurring from the necessity of having fixed metal frets on the modern classical guitar, which obligate it to play in equal temperament. Contrary to this habit, the baroque and renaissance guitar or lute used gut frets that were movable to compensate for these important peculiarities and problems arising from conflicting tuning practices.
How do these conflicting discrepancies affect the tone and timbre output of the modern classical guitar?
If for example we play the sixth string (E=82.4 Hz), it includes in its harmonic content the note g’# as fifth harmonic with frequency value: 82.4 x 5 = 412 Hz. The g’# found on the fourth fret of the first string, gives for its prime frequency 415.26 Hz derived by multiplying the first open string (e’=329.6 Hz) by 1.05946 and also repeating the procedure for each next frequency until the fourth fret is calculated.
Between the g’# (fifth harmonic of the sixth string) and the g’# (prime frequency found on the fourth fret of the first string) there is a noticeable audible difference in frequency of more than 3 Hz that creates beats at a rate of a little more than three full vibrations per second. Beat frequencies are created when two or more frequencies have a small difference in Hz value when sounded simultaneously.
This creates an audible distinguished harshness to the sound that is not pleasant when the notes are played simultaneously and this problem is carried on in all the frequencies of the instrument. If one adds the possible deviations from the ideal behavior of the harmonic spectrum that are inevitable to occur, meaning that the fifth harmonic partial of the sixth string might deviate on the specific guitar even more downwards in its response, than the problem of tone harshness of the instrument becomes even greater.
This beat frequency problem occurring between the conflicts of the harmonic partials in their simultaneous sounding with the prime frequencies of the same notes with occurring discrepancies cause harshness and also loss in the beauty of the tone. The cause for this acoustical and musical problem lies mainly in the relation that exists between the harmonic series behavior and the necessity of having a fixed fretted instrument.
Not few guitars, while they possess an outstanding sound in volume in general terms, however, present a harsh and unpleasant feeling when the beat frequency problem is there and it was not solved acoustically in the design of the construction of the instrument.
If this problem is not solved by the acoustic design of the reverberation chamber (the functional properties of the inside space of the instrument that will be a direct result of the defined chosen outline) it will remain a main disadvantage for the tonal and timbre qualities of the guitar.
The mathematical model of the guitar provides various solutions to this specific tonal problem that is pinpointed and specifically defined here and in the following article these solutions will be explained in detail.

Classical guitar constructed in 2010 by the author incorporating “The Kertsopoulos mathematical model of the guitar” with his innovational mechanism of the right hand pedal effect shown (left) and the overlaid with acoustical wooden stripes back and sides (right)
About the author: A classical-flamenco guitar soloist, composer, professor, luthier and string constructor, the Greek – Canadian Yorgos Kertsopoulos is also a researcher, inventor, author and founder of “the Guitar’s mathematic model and Geometric progression” (DAS MUSIKINSTRUMENT, Heft 9/ Sep. 1984, in German and English “The Physics of the Guitar”- Presentation of the same at the International Music Messe-Frankfurt, College of Furniture-London and seminars to respected luthiers and guitarists of England invited by GUITAR magazine)…. “His work is so unique in its scientific approach, but also so full of traditional truths, it includes everything…” -Jose Ramirez (at the press conference given by Y.Kertsopoulos at the Music Messe in Frankfurt-1983). His work as “Kertsopoulos aesthetics” involves the constructional revival of the different forms, tunings and sound timbres the guitar possessed in its history.
Note 1: The first known written definition of what is now called the golden ratio is provided in Euclid’s Elements (Greek: Στοιχεία): “A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the less”.
Note 2: Many studies of the Acropolis, including the Parthenon note that many of its proportions approximate the golden ratio, where the Parthenon’s facade and its elements are designed with the inclusion of golden rectangles.
Note 3: Mark Barr (20th century) suggests the Greek letter (φ), the initial letter of Greek sculptor Phidias’s name, as a symbol for the golden ratio.
Note 4: Very useful has been the (inverse) golden ratio also commonly referred as the golden ratio conjugate and denoted by the capital Phi (Φ): Φ = φ – 1 = 1.6180339887… – 1 = 0.6180339887…The first known approximation of the Φ was written in 1597 by Prof. Michael Maestlin in a letter to his former student Johannes Kepler.
Note 5: The golden section was used in the design of Notre Dame in Paris and continues today in many examples of art, architecture and design and used also in movements in the stock market.
Smaro Gregoriadou interpreting on a classical guitar respecting “The Kertsopoulos mathematical model of the guitar”:

Yorgos Kertsopoulos interpreting on a classical guitar respecting “The Kertsopoulos mathematical model of the guitar”:
Dionysia Blazaki and Yorgos Kertsopoulos interpreting on two classical guitars, both respecting “The Kertsopoulos mathematical model of the guitar”:
Review Entry 3 « artistuchtamatchu (13 years ago)
[…] http://guitarinternational.com/2011/09/05/the-golden-ratio-and-the-guitar-a-match-made-in-equal-temp… Share this:TwitterFacebookLike this:LikeBe the first to like this post. Categories: Uncategorized Comments (0) Trackbacks (0) Leave a comment Trackback […]
The Golden Ratio and Musical Aesthetics and Acoustics | musematics (11 years ago)
[…] One measurement of the Kertsopoulos model http://guitarinternational.com/2011/09/05/the-golden-ratio-and-the-guitar-a-match-made-in-equal-temp… […]
Golden ratio in guitar solos? | Uncommon Descent (10 years ago)
[…] writes to say that his high school guitar teacher told him that one can find the golden ratio in guitar solos that sneak into […]